Superconductivity: Difference between revisions
Aplstudent (talk | contribs) No edit summary |
Aplstudent (talk | contribs) No edit summary |
||
| Line 39: | Line 39: | ||
<h4>Lab Four: Determining the Critical Current Density </h4> | <h4>Lab Four: Determining the Critical Current Density </h4> | ||
By using a current source we will be able to measure the current densities as we make adjustments to the current source. We setting the current through the probe from a range of 0.1-0.5 Amps, data points were collected at its critical temperature. | By using a current source we will be able to measure the current densities as we make adjustments to the current source. We setting the current through the probe from a range of 0.1-0.5 Amps, data points were collected at its critical temperature. The approximate adjustment to the current resulted in a ratio of critical temperatures. | ||
[[File:Lab_Four_superconducting_systems.png]] | [[File:Lab_Four_superconducting_systems.png]] | ||
Revision as of 21:28, 14 June 2015
Superconductivity Project
Experiment Guide (Colorado Superconducting Kits)
As an introduction to superconducting system, three experimental guides were worked through to demonstrate different properties of super conductors. Below were the experiments conducted, before advancing to the mr. SQUID kit.
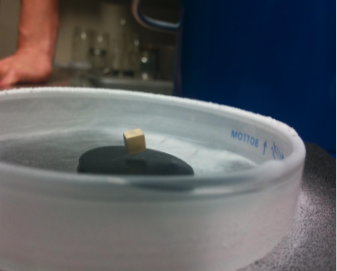
Lab One: Messner Effect
Definition: The expulsion of magnetic flux when a material becomes superconducting in a magnetic field. If the magnetic field is applied after the material has become superconducting, the flux cannot penetrate it.
The goal of this first lab is to show that this is true.
Observations: As the system dips below the critical temperature the magnet levitates. The magnet can then be sent into rotation, which in a perfect environment would rotate forever due to its frictionless property. The system increases in temperature due to the room, and will no longer levitate once it passes the critical temperature.
Lab Two: Resistance versus Temperature and Critical Temperature
As an extension to lab one, this experiment demonstrated the critical temperature of the system.
The critical temperature by measuring the Meissner effect was at 112 Kelvin.

Observations: As the temp begins to increase the magnet seems to float lower, as it reaches contact with the superconductor is when we know it has hit the critical point.
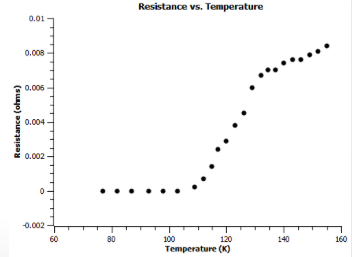
Lab Three: Measuring Resistance Versus Temperature and Critical Temperature
By measuring the electrical resistance as a function of temperature. We will have an insight to the Critical Temp, Critical Current Density and the Critical Magnetic Field.
Observations: The system remains constant initially as we submerge the the superconductor the voltage probe voltage read out abruptly increases. The system remains constant initially as we submerge the the superconductor the voltage probe voltage read out abruptly increases. The critical temperature was about 117 Kevin.
Lab Four: Determining the Critical Current Density
By using a current source we will be able to measure the current densities as we make adjustments to the current source. We setting the current through the probe from a range of 0.1-0.5 Amps, data points were collected at its critical temperature. The approximate adjustment to the current resulted in a ratio of critical temperatures.
Observations: The curve was extrapolated to 77 kelvin to find the Critical current. I(critical)=1.09 A.